Tag: Investing Page 1 of 5

Dilemma in selecting hybrid funds

On January 17, 2025

Since March 2023, the category of hybrid funds has witnessed a significant surge in assets under management (AUM), almost doubling. This is largely due to the favorable tax treatment of certain hybrid fund types and their ability to offer diversified exposure across different asset classes.

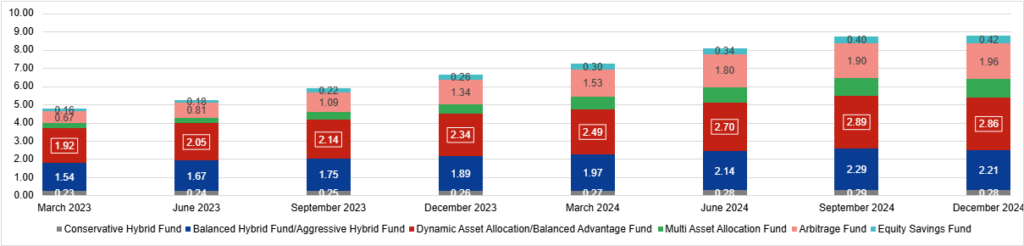
Source: Hybrid Fund AUM (Rs Lakh crore)(AMFI Data)

The rise in popularity of Dynamic Asset Allocation funds, Balanced Advantage Funds , Multi-Asset Allocation Funds, and Equity Savings Funds can also be seen as part of a broader trend where investors are increasingly seeking tax-efficient, diversified investment strategies, especially in the current volatile market environment.

However, investors often make mistakes when selecting these hybrid funds, primarily due to a lack of alignment with their individual risk profiles, financial goals, and other critical factors. Here’s a closer look at some of the common mistakes investors make when choosing hybrid funds and how they can avoid them:

Overestimating Stability and Underestimating Volatility :

Some investors mistakenly believe that hybrid funds, due to their diversified nature, are always low-risk. While hybrid funds are less volatile than pure equity funds, they can still experience significant fluctuations.

A Balanced Advantage Fund /Dynamic Asset Allocation Funds might have a large allocation to equities, which could lead to substantial volatility in bear markets. If an investor expects consistent returns, they could be caught off guard during periods of market downturns.

It’s important for investors to understand that while hybrid funds are designed to reduce volatility compared to pure equity funds, they are not completely risk-free. They can still be impacted by market corrections, interest rate changes, and other macroeconomic factors

Ignoring Fund Strategy and Asset Allocation:

Focusing too much on past returns without understanding the underlying asset allocation strategy of the hybrid fund can lead to poor decision-making. The asset mix and how actively the fund manager adjusts the allocation based on market conditions are crucial factors in determining the future performance of a fund.

Always check the fund’s investment philosophy and asset allocation strategy. This gives a clearer picture of what to expect from the fund beyond just past performance.

Time Horizon:

Hybrid funds, which invest in both equity and debt instruments, typically experience higher volatility than debt-only funds. As a result, these funds are better suited for medium to long-term investment horizons. While they aim to balance risk and return by adjusting the equity and debt allocation, hybrid funds are not immune to short-term market volatility.

For investors with a shorter investment horizon, liquid funds or arbitrage funds are more appropriate.

Comparing one fund with another:

One of the unique characteristics of hybrid funds is their flexibility to allocate between equity and debt based on the fund manager’s strategy and prevailing market conditions subject to SEBI MF Regulations. This flexibility means that hybrid funds are not directly comparable to each other in the traditional sense, especially when compared to more straightforward equity or debt funds.

A Dynamic Asset Allocation Fund may hold a higher percentage in equities during a bull market, while another may hold a lower percentage. Some hybrid funds have a higher equity exposure, targeting more aggressive growth over the long term. Others may maintain a lower equity allocation and balance with arbitrage opportunities, focusing on capital preservation with some growth potential.

The type of debt instruments used in a hybrid fund (e.g., government bonds, corporate bonds, or money market instruments) also affects its risk and return profile. A fund investing in higher-rated bonds will typically have lower risk and lower returns, while one investing in lower-rated bonds could have higher returns, but with a corresponding increase in risk.

Because of the flexibility in asset allocation and different investment strategies, hybrid funds are not easily comparable. When evaluating these funds, it’s important to focus on the investment objective, risk tolerance, time horizon, and management style rather than just comparing them based on past returns or a single performance metric. Each hybrid fund is designed to meet specific investor needs, and understanding the underlying strategy is key to making an informed decision.

Disclaimer: Views expressed herein are personal in nature and cannot be construed to be a decision to invest

Why we Overestimate the Odds of Finding Multibaggers in Smallcaps?

By Rukun Tarachandani

On November 17, 2023

In Uncategorized

In September’s FOF, I gave a presentation on the Opportunity and Challenges in Smallcap Investing. A recurrent question post the presentation was about how Smallcaps which have become Largecaps have generated a lot of wealth and consequently doesn’t it make Smallcaps attractive? Here’s a thought many share: When we look at the list of past multibaggers, we often find that many of those stocks started as Smallcaps. This leads some to infer that the probability of a smallcap becoming a multibagger is very high.

What does the actual data tell us? Let’s define a stock that offers a 30% Compound Annual Growth Rate (CAGR) over five years as a ‘multibagger’. Analyzing past data (from 2013 to 2018) from the top 500 stocks, we find that there’s an 11% chance of any stock becoming a multibagger. Focusing only on Smallcaps, that probability rises slightly to 13%. In other words, out of 250 Smallcap stocks, only about 33 might deliver such returns. Furthermore, there’s a 16% chance of a Smallcap stock declining by 50% over five years, compared to 14% for the broader stock group. This data suggests we might be greatly overestimating the allure of Smallcaps.

But the interesting question is why do we overestimate these odds? The answer lies in a fallacy most of us fall prey to – Base Rate Fallacy. What appears to us a high probability phenomenon actually turns out to be a low probability phenomenon.

To understand Base Rates, we need to understand Bayes Theorem; a cornerstone of probability theory. And for that we need some notation. At first glance, the mathematical notation may seem daunting, but trust me, it’s quite simple and intuitive.

P(A) : Probability of event A occurring
P(A | B) : Probability of event A occuring, given that B has occurred. In other words, if we know that B is true, then what is the probability of A

Now, Bayes rule states that:

Translated to plain terms: If you want to know the probability of A happening given that B has happened – P(A|B), you can figure it out by looking at:

P(B|A) – How often B happens when A happens,
P(A) – The overall likelihood of A happening on its own, and
P(B) – Dividing those by how often B happens in general.”

A common error is to confuse P(A|B) with P(B|A). This error is also called the Inverse Probability error

Now, with this context let’s evaluate the probability of smallcaps turning to large caps.
So let’s put this in notation. We know a stock is a multibagger and we believe many of these are smallcaps. When we look at past multibaggers we find that 60% of the multibaggers were smallcaps.

P (Smallcap | Multibagger) = 0.6

It might be tempting to take this 60% as the probability that a Small Cap would become a Multbagger. But as we will see that would be a major error in estimating probabilities. When you think about it, what we really want to know is if a given stock is a smallcap, what is the probability that it will become a multibagger i.e. P (Multibagger | Smallcap)

By Bayes Theorem :

Of the top 500 stocks, half are Smallcap. So we know the probability of smallcap – P(Smallcap) = 0.5

Also, of all the listed stocks we found that approximately 11% of stocks become multibaggers; so P(Multibagger) = 0.11

Now, we have the required probabilities to calculate the probability we want

P (Multibagger | Smallcap) = (0.6 x 0.11)/0.5 = 0.13 i.e. 13%

The reason for this low probability is that the overall base rate for a stock becoming a multibagger – P(Multibagger) is extremely low (11%) and that number dominates the probability of a smallcap becoming a multibagger. Our intuition makes us focus on P(Smallcap | Multibagger) but the number that influences the end probability much more is P(Multibagger). You can try different assumptions for P(Smallcap|Multibagger) and P(Multibagger) in the formula and what you will find is that so long as P(Multibagger) is very low, the end probability will also be low.

Once you understand and appreciate inverse probabilities, you will see them everywhere. For example: many studies and books focus on very successful companies and try to identify the traits common to them. Again this is actually an Inverse Probability problem and prone to Base Rate fallacy. By starting with a list of very successful companies and then identifying a common trait, we are finding P(Trait | Success). What we are actually interested in is P(Success | Trait) i.e. if a company has a given Trait then what is the probability that it will be successful.

How can we mitigate this fallacy? When probabilistic judgements, especially for low probability events, we need to take a step back and ask ourselves whether the Probability we have calculated or assumed is what is relevant to the decision or have we estimated an Inverse probability where we need to account for base rates. By ensuring that we critically evaluate the information at hand and avoid jumping to conclusions based on intuition or selective data, we can make more informed and rational decisions.

References:

This is a wonderful video to understand Bayes Theorem by the channel – 3Blue1Brown : https://youtu.be/HZGCoVF3YvM?si=KzIfHwyjZ32lx8HA

Games We Play

By Raunak Onkar

On July 15, 2020

In Investing

A few months ago I read a very nice post by @Alex_Danco on why VC’s should play bridge. It’s worth reading & it inspired me to write from my own experience of learning this interesting card game along with my friends, during the lockdown.

For any investor it is impossible to ignore these steps, the data, the analysis, the partnership, the expectation, the execution & finally luck.

In my view, the game of bridge is so well designed, that we get to see all these things play out in every single gameplay.

Do companies get the shareholders they deserve?

By Raunak Onkar

On May 9, 2019

In Behavioral Finance, Equity, Investing

Traditional Textbook Economics has the point of view of maximising utility. It means that each individual’s action in the economy is based on the expectation that they will make the maximum possible gain from a transaction. Whereas, behavioural economics has taught us, painfully, that we may not always be doing this well. We may set out with the best intentions of maximum gain but many times our actions fall short of the goal.

“It is not the final action, but the motivation underlying the action, that decides what is or is not viewed as cooperative or fair behaviour.”
– Games Indians Play by V Raghunathan

Thinking with Books #1

By Raunak Onkar

On June 20, 2018

In Investing

It’s an understatement to say that books have been instrumental in helping me to learn. Non Fiction Books not only provide some structure about the topic for the uninitiated reader but also provide an approach towards the topic.